We're trying to get our basement in order, and are finally getting rid of a huge old counter that was here when we moved in and is very inefficient storage. Putting in new cabinets, and a new smaller countertop (which we inherited) because we are a family that needs workspace.
So we're trying to figure out how big the shelves should be, and how we can arrange furniture in the new room. We've got some bunk beds that have to stay, but are quite awkward, and a small collection of other furniture. The upper right corner is a raised platform. OK to put things on, but they have to fit entirely on the platform. I wanted to make a scale image so we could move cutout furniture around, instead of just moving the real furniture around. "Hey!"it occurs to me, "I should make a dynamic sketch instead."
Onto Geogebra. I can always export the sketch for the paper version. My fifth grader helped me and we measured all the wall pieces and the bigger pieces of furniture. I made the furniture by making rectangles with fixed side lengths, with one side movable. Using the circle+radius tool, I discovered that you can put calculations in for the radius. So 4 feet 11 inches was entered as 4+ 11/12, or 30 inches as 30/12. (Yes I do know 30"=2.5', but the point is that conversion was easy.) Objects in Geogebra can be captioned, and in the properties-basic tab for an object you can choose to display nothing, name, value, name & value or caption as the label. Not too challenging to make, but a little tedious.
The sketch is a little too big to fit into the blog well. You can scale before exporting to make it smaller, but since you're moving furniture around, you want a little space. It's available as a webpage or as the original geogebra sketch. If you have any suggestions for it, please let me know. Or suggestions for the basement, for that matter!
Now get to work.
Update: this has been an interesting exercise. My 6th grader and her friend produced several possible layouts, my wife has used the geogebra for the first time, and my sister-in-law and father-in-law have also given it a spin. This has made some very accessible connections for people who do not see this stuff as math.
Also if you have a plan for the basement, my wife would also like to fit in a 52" round table. Thanks!
A blog for sharing my math interests on the web, to post new materials for elementary, secondary and teacher ed, and vent mathematical steam when needed. Thanks for visiting!
Pages
▼
Thursday, August 19, 2010
Wednesday, August 18, 2010
Teachers vs Athletes

VS.
Whose side are you on?
I know this is silly.
I love quotes, so I have quotes of the day popping up in all sorts of places. One today was:
"America believes in education: the average professor earns more money in a year than a professional athlete earns in a whole week." - Evan Esar"More like in a minute," I think. I tweet the quote and twask: Problem: how long does it take the average prof. athlete to earn the average annual teacher salary? Calling W|A! (Mr. Esar is listed in wikipedia as a humorist.)
@SethBurn asked back: "@mathhombre Are minor league baseball player's professional athletes? How about women in the WNBA? AHL hockey? MLS soccer?"
Hmmm. Admittedly I was thinking about MLB, NBA and NFL. Onto Wolfram|Alpha!
My first try was "average salary professional athlete" which got me the average US salary ($40,690/year). One of the suggestions was "athlete salary" which returned this data.
That's a lot of info. To see all that for the future searches, just click on the search terms, which will take you to the W|A search page.
"average teacher salary" finds for all US education, training and library occupations, $46,610/year. All right! Elementary teachers (a suggested search by W|A): $50,040/year for 1.54 million workers. Secondary teachers: for $52,450/year for 1.06 million teachers. It interpreted secondary to be highschool, so Middle School teachers: $50,630/year for 650,000 teachers. It occurs to me to ask for a comparison here: professional athletes vs teachers, which isn't much more informative.
So I calculate the average teacher salary (excluding special education and vocational teachers): (50040*1.54+52450*1.06+50630*.65)/(1.54+1.06+.65) = $50,944 as an average teacher salary.
What about the pro athletes? I think the original Wolfram Alpha figure is interesting. But what about the big time leagues?
Baseball: "average MLB salary" got the major league baseball teams, "average baseball salary MLB" didn't work (some results about the energy expenditures of playing baseball). Can't get it to give me the number. Finally googled it up: $2,944,556 in 2007, for 28 players*30 teams, 840 players; NFL $1.4 million in 2006 for 53 players * 32 teams, 1696 players; NBA $5,215,000 for 432 players. (The 30 teams can have up to 15, but not all do.) So the average major league professional salary: (2,944,556*840+1400000*1696+5215000*432)/(840+1696+432) = $2,390,000 for about 3000 professional athletes.
What about hockey? I am a die-hard Red Wings fan, so it breaks my heart, but I don't think the NHL is a major professional sport in the US anymore. And if the Wings are out, MLS is right out.
The original question involves breaking down by hourly wage. Which raises the question of how many hours an athlete works. I considered a lot of approaches, but ultimately decided that most pro athletes work year round now, considering conditioning, and long hours during a season. Maybe. So we'll consider the 50 weeks by 40 hours per week. About $1,195 per hour. $50,944/$1,195 is about 42.
Like I shouldn't have known the answer would be 42?
Anyhow, that's about a week. So congratulations on your estimate, Mr. Esar.
Monday, August 16, 2010
Making Magic
From The Red Pyramid, by Rick Riordan. Now that my kids are old enough to read real books, I find myself mostly reading what they recommend. Which is a lot of YA fiction. I love that this genre has appeared in time for them. Rick Riordan is the author of Percy Jackson, and this is the first book in a new (somewhat similar) Egyptian-themed series. It's a fun read. (But I miss JK Rowling.)"One learns by doing," Zia said. "This is not school, Sadie. You cannot learn magic by sitting at a desk and taking notes. You can only learn magic by doing magic."
But how about that quote? Doesn't it apply to everything? What can you learn by taking notes?
I want a powerful metaphor to start my classes this year that gets this across. At least gives students a chance to understand that they have been robbed. Misinformed. Abused. Neglected?
A recurring theme - at least since Dickens, and certainly post-Potter - is the child with a suboptimal life discovering that they have an amazing destiny. I think that appeals to us because it is, in fact, true. Without getting all religious here, I really believe that there's something each of us can do that no one else could do as well, or as opportunely, or where we could do it. One of the reasons I love teaching math is that, if learned, it opens doors, creates possibilities, and enables new choices.
Another recurring theme is that we find ourselves with new powers. Magic, demi-god stuff, athletic ability, spider-sense... but sadly it's often the result of genetics (Kal-El, Percy and Harry) or an accident (Spider-Man, Daredevil, ... Captain Underpants?) and less often the result of study. (Batman. It's always Batman.) Another thing I love about teaching math is that when students learn something they can literally do something that they couldn't do before. Even if it's something insignificant, like solve any quadratic equation that anyone could ever dream up. One of the reasons I love Potter is that being a wizard doesn't solve Harry's problems, it's the start of a whole new world of even-harder problems.
So I'm thinking that one of my fundamental teaching problems is how to communicate these ideas to my students. It's all muddled up with the growth mindset stuff, and deeply connected to the Equity Principle. What is a metaphor that will connect? What is the narrative? What experiences have they had with which I can connect? What experience can I provide from which they can draw? In a teacher education class, I think we can motivate through their profession - I've had some luck with that. Sometimes it works connecting with other learning. Athletes considering their sport. But in a pure content class, when the students are convinced they know what it means to learn or do math and they are just, almost totally, wrong?
My biggest successes so far have come with trying to convince the students that my real goals are to teach problem-solving and/or learning how to learn, and we're just using the math as a context for it. With these other subjects, they're willing to think it might be different. "I've never had a problem-solving class before. Must be what they're like."
This reminds me of the Robert Duke video that's making the rounds. He talks about how students only pay attention to what's assessed. And, somewhat more subtly, teachers only attend to what's assessed. And chances are, you are not assessing what you really want students to learn from you. He goes on to share a model of Whitehead that boils down to get your students doing the magic to learn magic. (His example is playing the drums, but...)
This is the Gene Krupa magic.
Didn't learn that in a lecture.
What is your model of math? Do you share it with your students? How?
I would love to hear about it, by email, by comment, by your blogpost... but please, share!
Friday, August 13, 2010
Math and Tech: Fri
Last day of school! Where's the joy? As Maria asked: "I know you're tired, but didn't you have fun? It's because you were learning!" If you're interested in the whole week of notes, try mccmath.
Friday Morning
Playing to Learn - Maria Anderson
Don't let me tell you, see for yourself. (Link goes to Maria's famous Prezi.) Other suggestions:
- If you assign a game, can ask them to play to a certain score. Or post a screenshot of their high score in the discussion board.
- A good game gets harder as you get better.
- Look for when the math is the game. Not just practice.
- Line Gem
- Factortris
- Manga High - several games with good content and game play, and superhigh production values. I signed up yesterday, and I've already gotten unsolicited emails asking to help me upload students name.
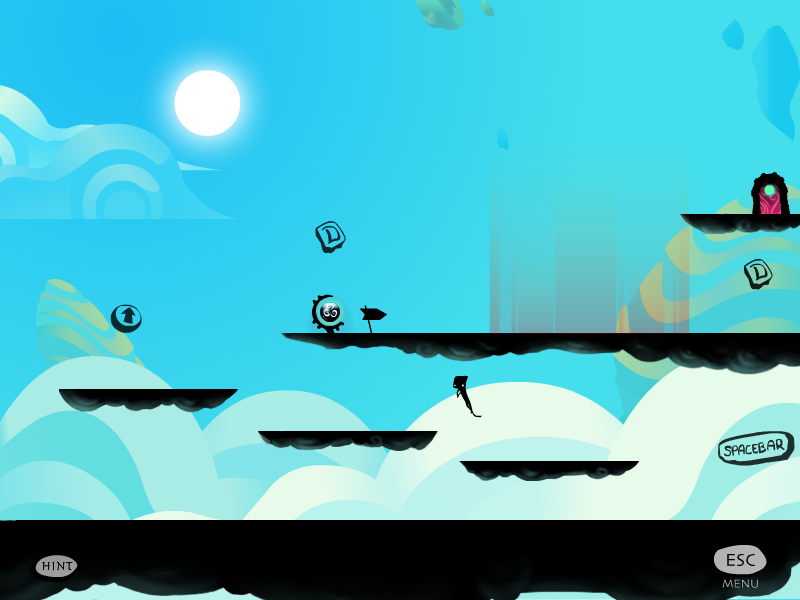
- Waker - this is a wicked fun game of graphing that also has nice situational problem-solving. (That's the screenshot at right.) Compare to Woosh. (Nice learning experiment: does narrative help? It must, right?)
- Jane McGonagol on gaming for a better world. (TED)
- Support the development of good math games. Say you'll use them, describe what makes the good ones good.
Friday Afternoon
Presentations. Our assignment: 5 min, 20 slides, (quick, how long per slide?) to share what we learned, resources to share, and our goals going forward.
My Prezi. Pretty lame for such a good week.
From the presentations:
- Big impact: things that allow us to share what we do. Jing, Squidoo, Mindomo, Mindmeister, Prezi, Google sites, blogging, ...
- Better life: Using tech to organize ourselves and improve efficiency. Eg. Using PBworks for class materials and not having to repeat them for each section/class, MathType, Symbaloo, Doodle, Tablet PCs and Wacom tablets, Soluto, Organize Your Digital Self (Maria's mindmap), Dropbox for sync, backup and storage, SpacedEd, ...
- New tools: Search-Cube, Cool Iris, Virtual Magnifier, SnagIt, Animoto, WinPlot, showmewhatswrong.com (Jing with no install), TodaysMeet.com, HootCourse (uses existing twitter and facebook accounts), Wolfram Alpha, Zoomerang, clickers, Camtasia, Online Stopwatch, Zotero research tool, bitly URL shortener, Vpython 3D python programming made easy (?), PrimoPDF free PDF maker, ...
- Fun: avatar editing (VOKI even), Imagination Cubed, ...
- Resources: Cyberchase activities, National Library of Virtual Manipulatives, Cool Text graphics generator, InterActMath a supplement to college textbooks, PhET simulations, ...
- Collaborate: Twitter, GALE, ...
- Goals: presentations, innovations in teaching, sharing with colleagues at home, establish online presence, ... In short, go forth and do stuff.
Was a good week. If it's open next year - you should consider it. We could all use a little change.
Thursday, August 12, 2010
Math and Tech: Thor's Day
Why do we have Norse-named days anyway? I want a cartoon here of Thor's screwdriver and pliers.
Wolfram|Alpha - Maria Anderson
Synchronous learning - Jill Mueller
Wiziq
Vyew
My gut reaction is that I'd use wiziq for one time or infrequent sessions, vyew for a regular gig. Students could bookmark your lobby, and make it dead easy to connect.
Open Session
Thursday Morning
Wolfram|Alpha - Maria Anderson
- Start playing with Wolfram Alpha. Enter line (1,2) (5,5). Wow. Notice what extra information there is. Maria set us the problem of how to graph a line with a point and a slope. (Took some work.)
- How to have students share: best in a chat room, where students can chat the cool entries that they find. Otherwise can use a discussion board. An interesting post is that the URL with your result will always bring up that exact same page you saw. Well, with the proviso...
- Wolfram|Alpha is updated weekly. If it can't do it now, try next week.
- Maria's favorite Wolfram Alpha posts.
- See the data available on a specific topic: here's my town - Grand Haven, nutrition, etymology of a word (Even shows rhymes! But not for orange.)
- Try comparisons: cities, companies, species (took some clicking on options to get this result), publications, ...
- Notice or beware that this works on smartphones. (Goodbye TI)
- Is W|A skynet?
- Have students use
SkynetW|A to factor. What do they notice? Connections with graphs? Can they generalize? - Careful: log(x) means natural log to W|A. lg(x) means log_10 (x)
- Find data they are interested in for applications, display, etc.
- Replace graphing calculators.
- On assessments, give them the graph or info they would have gotten from W|A. Now what can they do with it.
Thursday Afternoon
Synchronous learning - Jill Mueller
Wiziq
- Free. Do need to sign up. Anyone can be a teacher. Classes have whiteboard (with tools), audio, chat, ...
- Teacher turns on permissions for students: whiteboard, microphone, etc.
- Has some basic math symbols in the whiteboard typing.
- Compare to Elluminate
- Teacher can turn on screen sharing to show what's on for them. Very handy with MathXL or ... anything. Can't chat while sharing a screen.
- Non-teachers can share screen, but it shows up as a non-resizable window.
- Can monitor wiziq window and your screen share simultaneously.
Vyew
- Many similar features
- Create a lobby, and then you link students to there. Vs. creating a new link with each session in Wiziq.
- Don't have to register, you can just join.
- Teacher can set status of participants.
- There's a screen sharing tab, and it doesn't obscure the chat. (Not sure how the teacher will see the chat without switching back to the vyew window, which will then show itself, infinity, boom. )
My gut reaction is that I'd use wiziq for one time or infrequent sessions, vyew for a regular gig. Students could bookmark your lobby, and make it dead easy to connect.
Open Session
- Finish my Camtasia student film
- Start my required mini-presentation for tomorrow
- Work on mindmap on Conditions of Learning
- Google document to share participants' contact info (nice idea, Susan)
Wednesday, August 11, 2010
Math and Tech: Wed
Water heater went this morning so I missed some goodstuff. Sorry!
Presentation Design - Maria Anderson
Looks like they discussed topic generation, organizing potential slides and ideas with stickies, and the importance of visuals to go with presentation. Literally a visual for every point.
Some resources:
Camtasia is better than Jing for long format screencast videos, can record from screen and webcam, and has a fairly robust editor.
Editing tips
Should I put up my sample or not?
Wed Morning
Presentation Design - Maria Anderson
Looks like they discussed topic generation, organizing potential slides and ideas with stickies, and the importance of visuals to go with presentation. Literally a visual for every point.
Some resources:
- Maria's blogpost on designing digital presentations.
- Presentation Zen: 11 ways to use images poorly
- Garr Reynolds' Organization and Presentation Tips
- Slideology Blog and Book
- Sample Prezi on Levers of Change in Higher Education
- Prezi - groovy visual organizer for slides with very flexible positioning and sizing. Video imbeddable from youtube, or you can upload .flv.
- Animoto - turns a slide show into a video with some slick animation and a soundtrack. Easy to imbed, upload and share. Big trick: in Powerpoint, save as jpegs, then they will upload directly. Spotlight images you want for emphasis. Easiest to add text in PowerPoint. Can include video. Sadly, free accounts can only do 30 sec video, but with the educator account, you can give students access to your account.
- Mindomo , Mindmeister - mindmaps. (I might have to use Mindmeister just because of the Music Meister. Who is the Music Meister?) Mindomo is flash so no iPad or iPhone. Mindmeister is HTML5, but has slightly fewer features.
- What mode to choose? Consider: venue, audience, time, digital or not, and presentation style
- Venue: tech available? 1 computer per participant? Room setup? In a computer lab, go for interactivity; for example, mind maps.
- Content: lots of screenshots, consider just powerpoint. Full frame easy to see images.
- Completely digital: not very long, just want to get across a clear single point (eg. function notation)
- Impact: prezi to make a big impact. Levers of Change was for futurists, and a big wow was wanted.
Wednesday Afternoon
Camtasia Editing - Conan HeiseltCamtasia is better than Jing for long format screencast videos, can record from screen and webcam, and has a fairly robust editor.
Editing tips
- Export formats: Quicktime (.mov) for most purposes. Html creates a webpage with a player and a .swf file, but you need to be able to upload folders to make that work online.
- Control the speed of actions by their duration. Actions are transitions from one set of properties to another set. About a second as a rule of thumb, effects for a second. Adjust to make your point.
- Visual effects have meaning, whether you intend them to, or not. Fade is the most gentle. Say instead of a shift of seconds you want a shift of hours. Use a different effect to make your point.
- Types of effects are transitions, filters and actions.
- Smartfocus effect is an action that allows the camera to focus in on where your mouse travels. Smartfocus only works on Camtasia recordings, as they use extra data that was collected by the program.
- Filters apply in order. Sometimes this matters but often it doesn't. Example: color adjustment, then colorize is more effective. On the timeline, you can right-click, copy filters to new objects.
- Properties gearbox allows access to important information and control. But most things you can do through other tools. Exception: rotations of the input video.
- Custom video action does nothing by itself, but you can learn to use it to do all the transitions.
- To change aspect ratio hold down shift while resizing. Default is to preserve aspect ratio because it's video.
- Choose canvas size, add effects, organize... but do cuts last. They are the "most destructive" and the hardest to undo.
- Scripts are helpful. Ummm... minimize hesitations and... um.... keep you on flow.
- Storyboards: not for everyone, but can be very helpful.
- Instead of cutting a clip to get rid of verbal miscues, you can separate the audio and video, and just cut out the offending audio. You can also do that to keep audio you want without video. Cut out a corresponding bit of audio later, and realign the clips.
- Beware of video-noise. Use zooms and smartfocus (or the custom action to get that effect). Arrows to direct attention. (Turn arrows in the property box rather than rotate them parallel to the clip to make the gradient look right.) Window Spotlight blurs everything outside of a region set apart by contrast.
- Camtasia uses "tweening" with actions. Accelerates them in and decelerates them out, as opposed to having them happen with a constant speed. Can create some strange effects
by layering actions.
- Youtube: Choose Youtube or Youtube HD. Understand it is going to be shrunken down. Advantages - well known, great indexing for search, better for attracting followers.
- Screencast: can embed from screencast.com (TechSmith's videohosting site.) Advantages - doesn't reencode, so clearer, and more control. You can password protect it, make collections, only allow access to a single movie, etc. Screencast is where Jing stores video.
- Blackboard: might be better to use another sharing option then link.
- iPhone and iPad: export to .mp4
Should I put up my sample or not?
Fraction Multiplication
Meant to have this done for the most recent Math and Multimedia Carnival, but I just couldn't finish it. (Obviously still worth checking out the carnival, though.)
One of my goals for this week was to get fluent at embedding Geogebra in the blog. Kate Nowak already laid out the directions, so it should be easy peasy.
Fraction Multiplication - Area Model
In this picture, the grey rectangle represents one unit of area. Two fractions can be shown relative to that rectangle, as well as their product.
Can you see how the sketch shows the red fraction? The blue fraction?
If you uncheck show product, can you predict what it will be?
Can you see why the unsimplified product is what it is?
Does the simplified product make sense compared to the grey rectangle being equal to 1?
Created with GeoGebra
Also available as a standalone webpage or the original file. My only difficulty embedding was getting the first line of the applet correct. It needs to be as below, where the height and width are what's appropriate for sketch. (The archive value was without the web address in my html export from Geogebra.)
As for the Geogebra, here's what I did. Construct a rectangle, and measure the dimensions. Set up sliders for numerators and denominators, by limiting the range and setting step size to 1. Debated about allowing improper or not. When I decided to allow it, made the numerator sliders proportional, so the visual would support the comparison. I made the fraction rectangles by using the slider fraction to establish a proportion of the unit dimensions. So if the unit width was 9 units and the fraction was 2/3, made a circle with radius (2/3)*9. (literally (e/f)*distanceAC) The partition lines were made similarly, by making a unit distance and then translating the lines. The trickiest bit was the conditional visibility of the partitions. I set them to be visible if the the numerator was large enough. (I.e. the 12th line is only visible if the numerator is >= 12.) Then when I made the show/hide buttons, I lost all that. Sigh. I went back in and used the boolean variable and AND (^ from the menu, not the up carat which is for exponents), like w ^ j>=12.
I haven't ever had critical feedback on my sketches, so if you feel inclined, please let me know. (Well, students let you know, but I mean collegial feedback.)
OK, here's my Camtasia student film. I'm definitely interested in screencasts, but am not sure as to what features make them effective (or annoying), and how to use them effectively. This must be the most boring one, ever.
One of my goals for this week was to get fluent at embedding Geogebra in the blog. Kate Nowak already laid out the directions, so it should be easy peasy.
Fraction Multiplication - Area Model
In this picture, the grey rectangle represents one unit of area. Two fractions can be shown relative to that rectangle, as well as their product.
Can you see how the sketch shows the red fraction? The blue fraction?
If you uncheck show product, can you predict what it will be?
Can you see why the unsimplified product is what it is?
Does the simplified product make sense compared to the grey rectangle being equal to 1?
Created with GeoGebra
Also available as a standalone webpage or the original file. My only difficulty embedding was getting the first line of the applet correct. It needs to be as below, where the height and width are what's appropriate for sketch. (The archive value was without the web address in my html export from Geogebra.)
applet archive="http://www.geogebra.org/webstart/geogebra.jar" code="geogebra.GeoGebraApplet" codebase="./" height="560" name="ggbApplet" width="639"
As for the Geogebra, here's what I did. Construct a rectangle, and measure the dimensions. Set up sliders for numerators and denominators, by limiting the range and setting step size to 1. Debated about allowing improper or not. When I decided to allow it, made the numerator sliders proportional, so the visual would support the comparison. I made the fraction rectangles by using the slider fraction to establish a proportion of the unit dimensions. So if the unit width was 9 units and the fraction was 2/3, made a circle with radius (2/3)*9. (literally (e/f)*distanceAC) The partition lines were made similarly, by making a unit distance and then translating the lines. The trickiest bit was the conditional visibility of the partitions. I set them to be visible if the the numerator was large enough. (I.e. the 12th line is only visible if the numerator is >= 12.) Then when I made the show/hide buttons, I lost all that. Sigh. I went back in and used the boolean variable and AND (^ from the menu, not the up carat which is for exponents), like w ^ j>=12.
I haven't ever had critical feedback on my sketches, so if you feel inclined, please let me know. (Well, students let you know, but I mean collegial feedback.)
OK, here's my Camtasia student film. I'm definitely interested in screencasts, but am not sure as to what features make them effective (or annoying), and how to use them effectively. This must be the most boring one, ever.
Tuesday, August 10, 2010
Math and Tech: Tues
Previously: Monday
Framework: Pride-Teach
We experimented with controls in the plotting functions of Mathematica. Hard for me to attend to because its use is beyond many of my students tech skills, and the classes where it would be appropriate, we use Maple. Some people are exploring Geogebra instead. (Obviously, I'm down with that.)
Resources:
Tuesday Morning
Clickers/Classroom Response Systems: with Derek Bruff. Worthwhile even if you're not using clickers, because of the focus on pedagogy and student activity.- Near riot as we discussed: where does most of the mass of a tree come from: a) soil b) air c) water d) sunlight. "Right answer"= (b) because of carbon. (I dissent:
- Best clicker questions will provoke discussion. Had a nice teacher move flowchart.
- Many traditional systems. Also polleverywhere.com, and ResponseWare's smartphone/web solutions.
- Math questions like: You were once exactly 3 feet tall. T/F
- Can ask with confidence rating. i.e. Yes - High Confidence, Yes - Low Confidence, No - Low Confidence, No - High Confidence.
- Conceptual vs. Procedural questions - Both work. Key question: do answers allow students to work backwards from answers? (OK if that's intentional.) Can give them steps in the procedure and ask to find the error.
- http://derekbruff.com/teachingwithcrs/ - article on CRS are active and valuable assessment.
- http://mathquest.carroll.edu/resources.html - Sample clicker question bank.
- Create Time for Telling: make moments that create curiosity and the responses of peers matter to each student. Then they want you to tell them why or how. Motivationally they are ready. Often comes when most of the students are wrong.
- Can use for peer assessment. Students are hesitant to publically evaluate someone.
- Used for quizzes turns assessment into learning ops.
Framework: Pride-Teach
- Practicing or repeating
- Reading
- Internet
- Discussing
- Experiencing
- Thinking or reflecting
- Experimenting
- Academic
- Created
- Hearing or watching
- How do you learn? Evaluate 5 things you've learned recently with these standards. What do you notice?
- Started using projects to align how we expect our students to learn with how we learn.
- Cf. Marias el ed class: http://teachingcollegemath.com/?p=1632
- Cf. student presentations. Maria records feedback in Jing and gives video feedback. By the rubric that students have up front.
- These projects had beneficial effects on student performance on other assessments, and definitely addressed 21st century skills.
Tuesday Afternoon
Mathematica - PlotFest - Deb WoodWe experimented with controls in the plotting functions of Mathematica. Hard for me to attend to because its use is beyond many of my students tech skills, and the classes where it would be appropriate, we use Maple. Some people are exploring Geogebra instead. (Obviously, I'm down with that.)
Resources:
- Demonstrations from Wolfram, a huge collection of Mathematica Notebooks. These can be "played" using the free Mathematica player.
- Training videos that show some of the basic and advanced how-tos.
- Video of Deb talking about Mathematica
- Works well with Windows Journal or OneNote or Jarnal. (Jarnal's free and open source.) If you have a tablet PC you might want WinPenPal.
- Can use with Jing for helping students on WebAssign or MyMathXL, etc. because...
- Allows you to quickly write or jot math. One instructor at the session uses it to give notes, and then just posts the files online.
- It is easy to just drag images from wolfram-alpha, the web, or TI-Smartview to include in your handwritten notes.
- Free to create your avatar.
- Download the Second Life viewer.
- Set the rating of your account: General, Moderate, Mature, Adult. (Choose wisely.) Have to live 30 days before you can change more extreme than moderate, or by verifying with a credit card.
- Avatar names are unique. Common first names might be used up.
- Second Life Wiki
- Evert uses 2ndLife for discussions with people from all over. Better discussions with sense of close location. This can change with weird or distracting avatars, so ask your students to
- SlideShare pres on Math Ed in 2ndLife
- Search for freebies or swapmeet, teaching to find free gestures, like pointing.
- Can upload images, for ten Linden each. (Cheap but not zero.)
- Some of the math involved seems just to be in the design and manufacture of the sims. See this site for example. There's a free Math Sculptor.
- Any given moment there are 50-100K users; 2 million active accounts.
- Sounds like we should look up Janita Collins.
Monday, August 9, 2010
MCC Math and Tech Workshop
This week I'm at Maria Anderson's Math and Tech Workshop. I'll try to update this post as we go through the week. There's a large Explorers group and I'm in the Adventurer's group.
Learn more about this project
The end of the afternoon is working on Mathematica. It has developed quite a bit in the 15 years since I've last used it!
Monday Morning
Adventurers brainstormed the following for discussion. So look for more on this this week.- Wolfram Alpha
- Attention-Getters
- Textbooks: cost, open source
- Geogebra
- Vyew
- Student interaction
- Twitter/texting
- Math Ed Research on Tech
- Textbook cost: article in NYT
- Math Ed Tech Research: CITE Journal, which has different topic areas, including math.
- Use Facebook pages for students. They like the page and get those updates rather than your updates.
- Go over privacy settings with your students. They are often ignorant of the reality. Check your own.
- Linked In: limited use, like a modern rolodex.
- Twitter: internet equivalent of drinking from a firehose.
- Twitter is more collegial. Follow people who have worthwhile info to you, get nearly instantaneous feedback, hashtags to organize.
- Twapper Keeper (love that) to archive tweets.
- Twitter is a completely public space.
- Math Blogs: a node on Maria's MindMap of Spicing Up Mathematics
Monday Afternoon
Finally got time to play with Scratch. I'm wondering about it as an activity directly for students, for preservice teachers, or as an instructional design tool to make activities. Here's my first - kind of like pidgin Logo.The end of the afternoon is working on Mathematica. It has developed quite a bit in the 15 years since I've last used it!
Tuesday, August 3, 2010
Workshop and Responsibility
For a presentation at Michigan Council of Teachers of Mathematics 2010. It went pretty interestingly - teachers really focused on the idea of choice. There was a lot of interest in thinking about student responsibility. Quite a bit of interest in the parallel with learning language and the Conditions of Learning.
It's hard to believe that I haven't written more about workshop here. The idea is transferred from the workshop structure used in elementary literacy teaching. This presentation, though, is more about the importance of having a structure that aligns with your beliefs about learning, and how that supports students to take more responsibility for their own learning. I'm not big (nor is my co-presenter) on telling others how to do things, but we do want to share what we've been doing, why, and how it's working out.
It's my first Prezi, so... sorry! Handouts are after the prezi. (Direct link to the Prezi site if that's preferred.)
Main handout, includes our contact info and references, and 4th and 8th grade low stress sample workshops.
Overview of the Workshop Structure as we practice it, with some of our motivation.
Workshop Connections
Handouts with sample workshops
Anything I forgot? Just write me.
It's hard to believe that I haven't written more about workshop here. The idea is transferred from the workshop structure used in elementary literacy teaching. This presentation, though, is more about the importance of having a structure that aligns with your beliefs about learning, and how that supports students to take more responsibility for their own learning. I'm not big (nor is my co-presenter) on telling others how to do things, but we do want to share what we've been doing, why, and how it's working out.
It's my first Prezi, so... sorry! Handouts are after the prezi. (Direct link to the Prezi site if that's preferred.)
Main handout, includes our contact info and references, and 4th and 8th grade low stress sample workshops.
Overview of the Workshop Structure as we practice it, with some of our motivation.
Handouts with sample workshops
- In-class 1: Powerpoint Presentation on Lesson Planning
- In-class 2: Two of them: Patterns review and division algorithm
- Homework 1: Two of them: work on a project and a review assignment
- Homework 2: Two reading workshops
Anything I forgot? Just write me.
Monday, August 2, 2010
Elementary Homeschool
It has a been a couple of years since I really looked at what was available for elementary homeschoolers, but a recent question has me looking at this again.
The main focus has got to be curriculum, but that's a difficult question. Many of the pointedly homeschool curricula are drill and kill to the extreme. For example, one homeschooler who reported 132 practice problems per lesson. This post is not going to be for those who are looking for that.
What to teach?
The core standards are definitely the wave of the next decade. Many states are going to have exactly these as content objectives and most states will be close. There are still too many objectives, and too little attention to process, but it's a place to start. The NCTM standards are practically venerable, now, but still the best guide to worthwhile content that there is. The NCTM Focal Points would have made a better start to a common core, but still could help a homeschooler know what to center on.
Doing Math
The toughest thing for a homeschooler is the same as for a school teacher - shifting from a weak tea vision of math being grinding calculations to a rich frothy mug of math as an active way of thinking. The key to this transformation is best exemplified in the NCTM Process Standards. Center your time spent in mathematics with the student engaged in:
Curricula
My favorite curriculum, bar none, is Contexts for Learning Mathematics. Excellent activities, literacy integration, and student and teacher support. I use these materials with my preservice elementary teachers. Issues are price (though they are almost half off right now), and focus on number to the exclusion of geometry. So a little bit of supplementary materials might be needed. Strong on pre-algebraic thinking and reasoning though.
Second choice, or for the whole span of content objectives would be Investigations in Number, Data, and Space. There's an option for online access to games for practice, too. Unfortunately, it can be a daunting task teaching it for someone who might feel shaky on the math themselves. If you want quality activities, and are willing to explore along with your student(s), this might be the series for you. Unfortunately it is priced like college textbooks, $420 for a year's worth of teacher books. You can buy by unit to supplement another curriculum.
An interesting option is the relatively recent translation of the Japanese curriculum. They're available by grade level or the overview. It does not cover all of our objectives, because the Japanese have a more reasonable curriculum, but it definitely covers what's important. There are workbooks, too.
The cheapest option I've seen that has some value is the Jump Math program. It's a bit worksheet centered, so you would have to supplement activities and problem solving, but there's a try at conceptual focus and it's a start.
Sue Van Hattum thinks it's worth looking at the Mathematics Enhancement Programme (it's English) - and I'll be looking at it. (You can always trust the Math Mama.)
Resources
Good math teaching is the same wherever it happens - it's supporting worthwhile and significant student learning. My main advice would be to try to transfer from the areas where you feel your teaching is strongest. Science, reading, etc. What's making that work, and how would it look in math? Emphasize making sense. Don't you and don't let your student do anything just because. Connect with other teachers (blogging, commenting, or tweeting) and discuss your teaching.
The main focus has got to be curriculum, but that's a difficult question. Many of the pointedly homeschool curricula are drill and kill to the extreme. For example, one homeschooler who reported 132 practice problems per lesson. This post is not going to be for those who are looking for that.
What to teach?
The core standards are definitely the wave of the next decade. Many states are going to have exactly these as content objectives and most states will be close. There are still too many objectives, and too little attention to process, but it's a place to start. The NCTM standards are practically venerable, now, but still the best guide to worthwhile content that there is. The NCTM Focal Points would have made a better start to a common core, but still could help a homeschooler know what to center on.
Doing Math
The toughest thing for a homeschooler is the same as for a school teacher - shifting from a weak tea vision of math being grinding calculations to a rich frothy mug of math as an active way of thinking. The key to this transformation is best exemplified in the NCTM Process Standards. Center your time spent in mathematics with the student engaged in:
- Problem Solving. The big one. Working on finding answers to questions when you not only don't know the answer, but you also don't know how to find the answer. The trying and finding of methods to investigate is the heart of mathematics.
- Representing. Making, interpreting, translating between and choosing ways of showing and displaying mathematical information. Numbers, equations, functions, tables, charts, mind maps, written descriptions, pictures, diagrams, physical enactments, etc. Typically this is the biggest support to problem-solving, and one of the key means of differentiating in mathematics.
- Connecting. Seeing how what you know, mathematical information, and the real world relate and what they can tell us about each other. Many problem solving strategies boil down to
- Reasoning. Following connections in a particular direction (forward or backwards) or examining the strength of those connections. Answering why and how does this work questions. One framework for this is a) Making sense b) Conjecturing c) Arguing (which includes Proving).
- Communicating. Sharing or recording what you know. Strive to be clear, coherent, complete, correct and consolidated.
Curricula
My favorite curriculum, bar none, is Contexts for Learning Mathematics. Excellent activities, literacy integration, and student and teacher support. I use these materials with my preservice elementary teachers. Issues are price (though they are almost half off right now), and focus on number to the exclusion of geometry. So a little bit of supplementary materials might be needed. Strong on pre-algebraic thinking and reasoning though.
Second choice, or for the whole span of content objectives would be Investigations in Number, Data, and Space. There's an option for online access to games for practice, too. Unfortunately, it can be a daunting task teaching it for someone who might feel shaky on the math themselves. If you want quality activities, and are willing to explore along with your student(s), this might be the series for you. Unfortunately it is priced like college textbooks, $420 for a year's worth of teacher books. You can buy by unit to supplement another curriculum.
An interesting option is the relatively recent translation of the Japanese curriculum. They're available by grade level or the overview. It does not cover all of our objectives, because the Japanese have a more reasonable curriculum, but it definitely covers what's important. There are workbooks, too.
The cheapest option I've seen that has some value is the Jump Math program. It's a bit worksheet centered, so you would have to supplement activities and problem solving, but there's a try at conceptual focus and it's a start.
Sue Van Hattum thinks it's worth looking at the Mathematics Enhancement Programme (it's English) - and I'll be looking at it. (You can always trust the Math Mama.)
Resources
- A membership in NCTM gets you access to the standards and the journal of your choice. Probably the single greatest resource for learning about the math, the teaching of the math, and finding worthwhile activities. The Calculation Nation games are free to all, though.
- NRich. Best single source for math problems I know. Searchable by topic, filterable by grade level and challenge level.
- Let's Play Math. Terrific blog of Denise, a homeschooling mother. Resources, links, activities, discussion. Also maintains a collection of Mostly Free Math Resources.
- Homeschool Math is run by Maria and is also worth a look.
- Me - write with questions and I'll help however I can.
Good math teaching is the same wherever it happens - it's supporting worthwhile and significant student learning. My main advice would be to try to transfer from the areas where you feel your teaching is strongest. Science, reading, etc. What's making that work, and how would it look in math? Emphasize making sense. Don't you and don't let your student do anything just because. Connect with other teachers (blogging, commenting, or tweeting) and discuss your teaching.
















